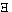HOME TOP UP PREV NEXT 0 1 2 3 4 5 GERMAN MAP      Tractatus Logico-Philosophicus 5.53

5.53

Identity of the object I express by identity of the sign and not by means of a sign of identity. Difference of the objects by difference of the signs.

5.530

5.531

I write therefore not "f(a, b) . a=b" but "f(a, a)" (or "f(b, b)"). And not "f(a, b) . ~a=b", but "f(a, b)".

5.532

And analogously: not "(x, y) . f(x, y) . x=y", but "(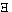x) . f(x, x)"; and not "(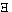x, y) . f(x, y) . ~x=y", but "(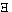x, y) . f(x, y)".

Therefore instead of Russell's "(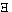x, y) . f(x, y)" : "(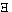x, y) . f(x, y) .v. (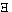x) . f(x, x)".)

5.533

The identity sign is therefore not an essential constituent of logical notation.

5.534

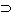And we see that the apparent propositions like: "a=a", "a=b . b=c . a=c", "(x) . x=x". "(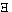x) . x=a", etc. cannot be written in a correct logical notation at all.

5.535

So all problems disappear which are connected with such pseudo-propositions.

This is the place to solve all the problems with arise through Russell's "Axiom of Infinity".

What the axiom of infinity is meant to say would be expressed in language by the fact that there is an infinite number of names with different meanings.